It sometimes seems like there is a pre-medical student everywhere you turn at UAB. Pre-meds are one of the most motivated (and sleep-deprived) groups of students on campus. The pre-med curriculum expects students to be very well-rounded and is not easy by any standard. These students take diverse and often difficult classes including general and organic chemistry, general physics, biology, and English literature. However, from my experience being friends with and tutoring many pre-med students, the subject they seem to dislike most is math—specifically calculus and statistics. One of the most common complaints I hear regarding these fields of math is “Why do I need to learn this? When am I ever going to use this as a doctor?”. I’d like to take the time to answer these questions for all of the pre-meds wondering the same thing, and present an argument for why upper-level math classes (past Calculus I and Statistics) are useful in medicine.

Author: David Chasteen-Boyd

It sometimes seems like there is a pre-medical student everywhere you turn at UAB. Pre-meds are one of the most motivated (and sleep-deprived) groups of students on campus. The pre-med curriculum expects students to be very well-rounded and is not easy by any standard. These students take diverse and often difficult classes including general and organic chemistry, general physics, biology, and English literature. However, from my experience being friends with and tutoring many pre-med students, the subject they seem to dislike most is math—specifically calculus and statistics. One of the most common complaints I hear regarding these fields of math is “Why do I need to learn this? When am I ever going to use this as a doctor?”. I’d like to take the time to answer these questions for all of the pre-meds wondering the same thing, and present an argument for why upper-level math classes (past Calculus I and Statistics) are useful in medicine.

The more immediate reasons that math is useful to future physicians are evident in the pre-med curriculum itself. First, many medical schools require either calculus or statistics (or both) to be considered for admission. The University of California at Berkeley Career Center has compiled a list of medical schools in the U.S. and Canada and their admissions requirements in different subject areas.¹ Out of 64 schools whose requirements are given, 40 schools require—and the remaining 24 recommend—at least one semester of college-level math. Some medical schools specified math courses that they want incoming students to have taken. Twenty-six of the schools recommend or require that students take calculus and nine of the schools require or recommend statistics. So, just to get into medical school, pre-meds frequently have to take calculus or statistics.

Additionally, almost every medical school requires students to take physics as well as general and organic chemistry, not to mention the fact that physics is well represented on the Medical College Admission Test (MCAT). These fields are strongly calculus-based. Physics is almost completely derived from calculus, and calculus was actually developed by Isaac Newton to further the study of physics. In a post on studentdoctor.net, a prospective doctor asked about the usefulness of math for physicians. Many explanations were given, but the general consensus was that an understanding of calculus leads to better comprehension of chemistry and physics.² Given that these subjects constitute a significant portion of the MCAT, it would benefit any pre-med student to learn calculus.

From a more abstract perspective, calculus offers new ways of thinking that are quite useful to a medical practitioner. Calculus is the study of both the infinitesimally small and the infinitely large. Differential calculus studies the very small; derivatives look at the behavior of a function at two points that are very close to each other and how that function changes between those two points (or more simply, derivatives are a way to represent a rate of change). This forces one to consider what kinds of changes happen on an infinitesimally small scale. These kinds of changes are common in biology and medicine; tiny changes in medicine (such as pH, drug concentration, etc.) can effect large changes in the health of a patient. Integral calculus (and the more general field of infinite series) is concerned with the very large; integrals are based on adding up an infinite number of small pieces to yield a bigger picture. In medicine, this leads to the ability to think of the body as the sum of many smaller pieces—namely the different systems, organs, tissues, etc.—and to consider their effects. Overall, the study of variations and how different variables changing can change an overall system has useful applications in medicine. This is exactly the function of calculus. For example, calculus was used to develop the Cockroft-Gault equation, which determines the appropriate drug dosage for patients with certain kidney diseases based on the level of creatinine in their blood. Equations like this are invaluable to physicians and could not exist without an understanding of calculus.³

In addition to Calculus I and II, there are many higher-level math classes that are useful in the study of medicine, especially to those who wish to conduct medical research. Two of these are multivariate calculus and differential equations. Multivariate calculus (called Calculus III here at UAB) studies the application of derivatives and integrals to functions of more than one variable. The dependent variable, z, can depend on two independent variables, x and y (but this can be easily expanded to a function that depends on any number of variables). For example, one could study the stability of a novel drug delivery system (dependent variable, z) changes in response to variations in pH and temperature (independent variables, x and y). Multivariate equations are more representative of reality, and they force one to consider the effects of many different variables on a variable of interest. It is rare in medicine to find a factor that depends solely on a single variable.  To consider a practical application of this manner of thinking, it has been shown that high stress in individuals can weaken the immune system.  So, stressed individuals can be more likely to get sick.  If a patient comes into a clinic with something as generic as the flu, doctors may need to consider treatments for causes other than just the virus that is infecting their patient.  Counseling or other stress-reduction techniques may also improve the patient’s long term health.  Seeing how multiple variables are contributing to a particular situation can be a useful skill for clinicians.

Differential equations involve equations that contain both a function and its derivatives. One of the simplest differential equations is displayed below.

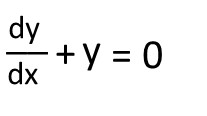
This kind of equation may at first seem trivial. What is so important about an equation that contains a derivative? It turns out that almost every real-world system can be modelled by differential equations. For example, the FitzHugh-Nagumo equations (and the more complex Hodgkin-Huxley equations) are a system of differential equations that are used to model the depolarization of neuronal or cardiac cell membranes.⁵ The developers of the Hodgkin-Huxley model were awarded the Nobel Prize in Physiology or Medicine for their work on the equations.⁶ These equations are useful to medical researchers because they can be used to study the function of cardiac cells under different stimuli, and by extension, to study the mechanisms of certain heart diseases.

While calculus has many more applications in medicine that could be discussed, it is also important to discuss how other fields of math are useful to physicians. Going back to high school, an understanding of algebra and proportions, known as functional numeracy, is vital to practicing medicine. Doctors prescribe drugs on a daily basis, and there is no one-size-fits-all dose of a particular drug. Doses are based on many factors, such as a patient’s weight, BMI, health conditions, etc. Calculating the correct dosage can involve determining the flow rate of a drug that is delivered intravenously, how often a patient should take a certain pill, and how large of a pill to give the patient. These questions can be answered using algebra and ratios based on standardized per-weight formulas.⁴ Additionally, experience with math (and physics and chemistry) leads to an understanding of what results are reasonable. If you have taken physics and chemistry, you have probably calculated an absurdly high or low number as the solution to some problem. You likely had a gut feeling that the answer was wrong and double-checked your work. Experience in the field can lead to an intuition for the reasonability of an answer, and practice problems in math (and other) classes may bolster one’s level of experience.⁷

Statistics is applied to every area of the sciences, including medicine. Research is constantly being done into many different aspects of medicine, and as a result the practice of medicine is constantly adapting based on emerging research. In order to understand—and more importantly, critically analyze—the results and data analysis sections of medical research manuscripts, physicians need a strong background in statistics. It is not uncommon for medical research manuscripts to report improper statistics, unsupported conclusions, and incorrect study designs.⁸ A deep understanding of statistics is necessary for physicians to identify well-designed studies and properly analyzed data. With this skill, a physician can identify which manuscripts contain data convincing enough to cause them to change their practice of medicine, and which conclusions are the results of poorly designed studies or inappropriate data analysis.

An understanding of calculus is also useful for analyzing the processes used to gather and filter data in medical manuscripts. Additionally, knowledge of these techniques can aid in the design and improvement of clinical studies. Dr. Mary Tai published a manuscript that described a “novel” method of measuring the area under a glucose tolerance curve.⁹ Glucose tolerance tests are used to diagnose conditions such as diabetes, insulin resistance, and others related to insulin metabolism. The area under a glucose tolerance curve is a useful measure of glucose excursion.¹⁰ Dr. Tai’s proposed formula for calculating the area under a glucose tolerance curve (which she named Tai’s method) involves taking the curve, splitting it into an appropriate number of intervals, and approximating the area in one interval as a trapezoid where the height of the trapezoid on either side of the interval is equal to the value of the glucose tolerance curve on either side of the interval. Anyone who has taken calculus will recognize this as the trapezoid rule for approximating definite integrals, which has been in use since as early as 350 BCE.¹¹ In other words, Dr. Tai reinvented a well-known numerical integration method, believing it to be a novel concept. She also validated this method by comparing the results of using her method to a “standard” area of the curve; she obtained her standard by drawing the curve on graph paper and counting the number of squares that were underneath the curve. This is clearly an inaccurate method of creating a control. Though this anecdote is amusing, it also serves as an example of why understanding calculus and statistics is vital to physicians, and especially to medical researchers. Other examples of calculus-based methods used to analyze medical data include image processing methods used to remove unwanted noise and objects from medical images and the Fourier transform, which can remove noise from ECG and other signals.

Calculus and statistics are used in the medical profession in fields ranging from measuring kidney function to analyzing medical images to diagnosing diabetes. Clearly, mathematics is vital to the medical profession. A deep understanding of mathematics will improve a pre-medical student’s performance in undergraduate science classes and on the MCAT.  Additionally, having a familiarity with these fields of math will improve his or her practice of medicine post-medical school.

References

1. University of California at Berkeley Career Center. “2015 Medical School Math, English, Biochemistry & Psych/Soc Requirements.” (2015): Web. 21 Dec. 2016
2. “Do Doctors have to be Good in Math????” (2000-2015): studentdoctor.net. Web. 23 Dec. 2016.
3. “Cockcroft-Gault Formula.” Kidney.org. The National Kidney Foundation. Web. 23 Dec. 2016.
4. Natasha Glydon. “Medicine and Math.” Mathcentral.uregina.ca. Math Central. Web. 22 Dec. 2016.
5. Hodgkin, A. L., and A. F. Huxley. “A Quantitative Description of Membrane Current and Its Application to Conduction and Excitation in Nerve.” The Journal of Physiology 117.4 (1952): 500–544. Print.
6. "The Nobel Prize in Physiology or Medicine 1963". Nobelprize.org. Nobel Media AB 2014.Web. 24 Dec 2016. http://www.nobelprize.org/nobel_prizes/medicine/laureates/1963/
7. Neil J. Nusbaum, “Mathematics Preparation for Medical School: Do All Premedical Students Need Calculus?” Teaching and Learning in Medicine, 18(2), (2006): 165–168.
8. Strasak, Alexander M.; Zaman, Qamruz; Pfeiffer, Karl P.; Göbel, Georg; Ulmer, Hanno.“Statistical errors in medical research – a review of common pitfalls.” Swiss Medical Weekly. 137 (2007): 44-49. Web. 23 Dec. 2016.
9. Tai, Mary M. “A Mathematical Model for the Determination of Total Area Under Glucose Tolerance and Other Metabolic Curves.” Diabetes Care. 17(2). (1994): 152-154. Web. 22 Dec. 2016.
10. Sakaguchi, K., Takeda, K., Maeda, M. et al. Diabetol Int (2016) 7: 53.doi:10.1007/s13340-015-0212-4
11. M. Ossendrijver. “Ancient Babylonian astronomers calculated Jupiter’s position from thearea under a time-velocity graph.” Science (2016). Web. 23 Dec. 2016.